(M-11a) Trigonometry Proficiency Drill

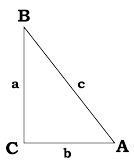A triangle ABC has a right angle C and two sharp angles A and B. The triangle's sides AC and BC on both sides of the right angle C are given as:
(a) AC = 3     BC = 4
(b) AC = 5     BC = 12
(c) AC = 8     BC = 15


In each case, use the theorem of Pythagoras to find the third side and then find the sine and cosine of angles A and B.

1. You are traveling uphill on a road and see a sign telling you this is a 5% grade, i.e. rising 5 meters for every 100 meters of road. What is the angle between the road and the horizontal direction?

   

2. An airplane is flying at 170 km/s towards the north-east, in a direction making an angle of 52° with the eastward direction.

   The wind is blowing at 30 km/s towards the north west, making an angle 20° with the northward direction. What is the actual "ground speed" of the airplane, and what is the angle A between the airplane's actual path and the eastward direction?

(The solution follows below: read it only after you have worked out the problem yourself. Teachers in class may substitute different numbers and directions)

Let us denote the velocity vector of the airplane relative to the air as V, that of the wind relative to the ground as W, and the velocity of the airplane relative to the ground U=V+W, where the addition is one of vectors. Draw a diagram with the given speeds and angles labeled appropriately.

To perform the actual addition each vector must be resolved into its components. We get

Add:

Therefore

4. In a triangle ABC, we name the angles (A,B,C) according to their corners ("vertices," singular vertex), and name the sides (a,b,c), so that side a is facing angle A, b faces angle B, and c faces angle C. Prove the "law of sines"
   sinA/a = sinB/b

   Hint: From C draw a line CD perpendicular to side c. The line CD is a "height" of the triangle and will therefore be denoted by the letter h. Use h in your proof.

5. Before attempting the next problem, note two points:

• If in the above proof we had used a perpendicular "height" from A or B, rather than from C, we would have got a relation like
  sinA/a = sinC/c

  Therefore the "law of sines" is completely symmetric:

  sinA/a = sinB/b = sinC/c

  

• The sum of angles in a triangle is always 180 degrees. A rigorous proof takes some work, but the statement can be made plausible by the following argument. In the triangle ABC, draw a line through point C that is parallel to AB. This creates two additional angles, A' and B'.

  The three angles (A',C,B') add up to 180 degrees, because they are adjacent to each other and backed off against a straight line. However, by the properties of parallel lines, the angles (A,A') are equal, as are (B,B'). Therefore (A,C,B) also add up to 180 degrees.

Don't read any further until you have tried to solve it. Teachers in class may substitute different numbers and directions.

Multiply both sides by sin(55) to obtain the length b = AC.

Further question: what is the perpendicular distance from C to the line c = AB? (hint: it equals the height h in the derivation of problem (4).)

6. (after section M-10) Find the sine and cosine of
   (1) 145°         (2) 210°         (3) 300°

   

7. (a) When a beam of light hits the surface of a flat piece of glass, it is generally bent by some angle. Draw a line perpendicular to the point on the surface where the beam enters. Then if the beam reaches the surface along a path making an angle A with the surface, it continues inside the glass with an angle making an angle B, where
   sin B = (sin A)/n
   The number n ("refractive index") is a property of the glass and is larger than one.

   The problem: given values of A=0, 20, 40, 60, and 80 degrees, and n = 1.45, what is B in each case?

   (b) the same rule holds when the light leaves the glass, except that now the angle B (inside the glass) is given and the angle A (in air) must be found. We then use

The problem:Given B = 0, 20, 90, 60, 80 --what are the angles A? Does any of these beams fail to leave the glass?

(By the way: the reason a prism splits light is that the value of n depends slightly on color of the light--or more accurately, on its wavelength).

8. In a set of cartesian coordinates the point P has coordinates (x,y), and as shown in the drawing, x = OA, y = PA.

   A second system with the same origin O has axes (x',y') formed by rotating the (x,y) axes clockwise by an angle a. In the same drawing, the new coordinates of P are x' = OB y' = PB.

   Using the auxiliary points (C,D,E) and the auxiliary lines AE and AD, express x' and y' in terms of x, y, sina and cosa.

   Hint (follow this step by step in the drawing). The two triangles CBO and CPA are right angled, and since the sum of the angles in a triangle is 180°, the two sharp (acute) angles in each of them add up to 90°. Of those sharp angles, the two that meet at C are equal, and therefore the other two must be equal, too.
       Of those other anglesAOB is given to be equal to a. Therefore the angle APC also equals a, as marked. Try to solve the problem before continuing.

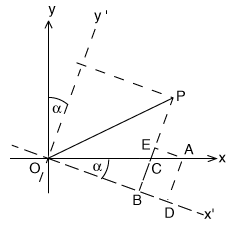
Remember: in a right-sided triangle with angle a, if you multiply the long side

• by cosa, you get the side near to a
• by sina, you get the side opposite to a

Let us rewrite the final result:

Algebra drill: using the above two relations, can you express (x,y) in terms of (x',y')? You must use sin²a + cos²a= 1.
Show also that the result is consistent with the first formula, if we exchange the roles of (x,y) and (x'y') and replace the rotation angle with (-a).

That would also agree with the figure, in that that (x,y) are obtained by rotating (x',y') counterclockwise by an angle a, which may be viewed as a clockwise rotation by an angle (-a).
        Click here for further discussion of this result, especially for those who have studied section #12A "How Orbits are Calculated."

9. How long is the line of latitude L if the Earth is a sphere and the equator-to-pole distance is 10,000 km? How long is one degree of longitude at latitude L?
       Does the formula also hold for southern latitude?

10. (a) If cos X = 2 sinX, what is sin X? What is the angle X?
    (b) Is this the only solution?
        Hints:
    (a) Use cos²X = 4 sin²X
    (b) Since we work with an equation that only involves squares, any solution of it only stipulates sin²X and cos²X. However, the original condition also requires that cos X and sin X have the same algebraic sign.